Answer:

Explanation:
To find the coordinates of endpoint B, we can use the midpoint formula. The midpoint formula is given by:

In this case, the coordinates of A are
 and the coordinates of R (the midpoint) are
and the coordinates of R (the midpoint) are
 . The coordinates of B are
. The coordinates of B are
 .
.
Let's use the midpoint formula to solve for
 :
:
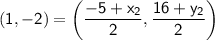
Now, we can solve for
 and
and
 :
:
1. Solve for
 :
:
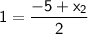
Multiply both sides by 2:
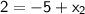
Add 5 to both sides:
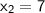
2. Solve for
 :
:
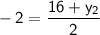
Multiply both sides by 2:
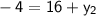
Subtract 16 from both sides:
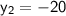
So, the coordinates of the endpoint B are
 .
.