Question

Find the mistake in the question and explain. show work with correct answer

Answer 1

Question 9: The given polynomial is
`\(f(x) = x^3 + 4x^2 + 9x + 10\)`, and the roots are provided as
`\(x = -2, -2 \pm √(8i)\).`

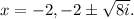
The mistake in the solution is in the synthetic division step. The correct synthetic division should be:

1 4 9 10

[-2] | -2 -4 -10

--------------------------

1 2 5 0

So, the correct quadratic equation is
`\(x^2 + 2x + 5 = 0\)`. To find the roots, you can use the quadratic formula:

`\[ x = (-b \pm √(b^2 - 4ac))/(2a) \]`

For this equation,
`\(a = 1\), \(b = 2\), and \(c = 5\).` Plugging in these values:

`\[ x = (-2 \pm √(4 - 4(1)(5)))/(2(1)) \]`

`\[ x = (-2 \pm √(-16))/(2) \]`

`\[ x = (-2 \pm 4i)/(2) \]`

`\[ x = -1 \pm 2i \]`

So, the correct roots are
`\(x = -1 + 2i\) and \(x = -1 - 2i\).`

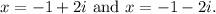
Question 10: The given solutions are
`\(4, 5i, -5i\)`, and the provided polynomial is
`\(x^3 + 4x^2 + 25x + 100\).`

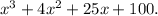
The mistake in the solution is in the factorization step. The correct factorization should be:

`\[ (x + 4)(x - 5i)(x + 5i) \]`

Multiplying these factors correctly:

`\[ (x + 4)(x^2 + 25) \]`

Expanding this expression gives:

`\[ x^3 + 4x^2 + 25x + 100 \]`

So, the given polynomial
`\(x^3 + 4x^2 + 25x + 100\)` is correct and matches the provided solutions. There is no mistake in this part.