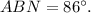Question

Find the measurement indicated in each parallelogram.

7. C
CE=5x+2
CB=6x+8
Find EB.
A
E
D
B
8.
N
B
G
L
Angle BAL= 86.
Find measure of angle ABN.

please helpppp

Answer 1

In the first parallelogram ABDC, solving for x reveals EB = -14.

In the second parallelogram BALN, ABN is
`\(86^\circ\)` due to opposite angles in a parallelogram.

First Parallelogram ABDC:

In parallelogram ABDC, CE is a diagonal with midpoint E, and CB is another diagonal. We are given that CE = 5x + 2 and CB = 6x + 8.

Since E is the midpoint, CE = CB.

5x + 2 = 6x + 8

Solving for x:

x = -6

Now, we can find EB, which is the other half of CE:

`\[EB = (CE)/(2) = (5x + 2)/(2) = (5(-6) + 2)/(2) = -14\]`

Second Parallelogram BALN:

In parallelogram BALN, G is the midpoint of the diagonal. The angle
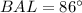`\(BAL = 86^\circ\)`, and we want to find angle ABN.

Since opposite angles in a parallelogram are equal, angle
`\(BAL = \angle BAN\).`

Therefore,
`\(ABN = 86^\circ\).`