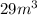Question

Help me i don’t know how to do it please answer

Answer 1

The total volume of the greenhouse, rounded to the nearest cubic meter, is 29 cubic meters.

V=
`29m^3`

To solve the volume of the greenhouse depicted in the image, we need to consider it as a combination of simple geometric shapes and calculate the volume of each, then sum them up.

The greenhouse can be divided into three parts:

1. A rectangular prism (the base part):

- Length (l) = 13 m

- Width (w) = 0.8 m

- Height (h1) = 0.8 m

2. A cuboid (the middle part):

- Length (l) = 13 m

- Width (w) = 0.8 m

- Height (h2) = 1.6 m

3. A triangular prism (the top part):

- Base length (b) = 13 m

- Width (w) = 0.8 m

- Height (h3) = 0.8 m

The volume of the greenhouse,
`\( V \)`, is the sum of the volumes of these parts:

Volume of the rectangular prism:
`\( V_(rect) = l * w * h1 \)`

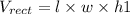
Volume of the cuboid:
`\( V_(cuboid) = l * w * h2 \)`

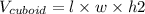
Volume of the triangular prism:
`\( V_(tri) = (1)/(2) * b * w * h3 \)`

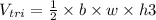
So, the total volume is:

`$$ V = V_(rect) + V_(cuboid) + V_(tri) $$`

Now, we can calculate each part:

`\( V_(rect) = 13 * 0.8 * 0.8 \)`

`\( V_(cuboid) = 13 * 0.8 * 1.6 \)`

`\( V_(tri) = (1)/(2) * 13 * 0.8 * 0.8 \)`

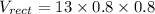
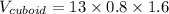
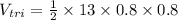
The total volume of the greenhouse, rounded to the nearest cubic meter, is 29 cubic meters.

V=
`29m^3`