Question

Find the area A of the sector shown in each figure

Answer 1

64

Explanation:

`S=(r^2\alpha)/(2)=(16^2\cdot 0.5)/(2)=64`

Answer 2

64

Explanation:

To find the area (A) of the sector of a circle where the central angle is measured in radians, we can use the area of a sector formula:

`\boxed{\begin{array}{l}\underline{\sf Area \;of\; a\; sector}\\\\A=\frac12 r^2 \theta\\\\\textsf{where:}\\\phantom{ww}\bullet\;\textsf{$A$ is the area.}\\ \phantom{ww}\bullet\;\textsf{$r$ is the radius.}\\ \phantom{ww}\bullet\;\textsf{$\theta$ is the angle measured in radians.}\end{array}}`

In this case:

• r = 16
• θ = 0.5 rad

Substitute the values into the formula and solve for A:

`\begin{aligned}A&=(1)/(2) \cdot 16^2 \cdot 0.5\\\\A&=(1)/(2) \cdot 256 \cdot 0.5\\\\A&=128 \cdot 0.5\\\\A&=64\end{aligned}`

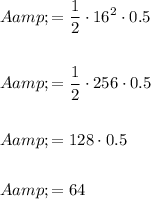
Therefore, the area of the sector is 64.