Final Answer:
The binomial series expansion of √(4-9x) |x| up to and including the term in x² is
 . Using this expansion, when x =
. Using this expansion, when x =
 , an approximate value for √(310) is 17.605.
, an approximate value for √(310) is 17.605.
Step-by-step explanation:
To find the binomial series expansion of √(4-9x) |x|, we start with the binomial expansion formula:
 . Here,
. Here,
 for the square root.
for the square root.
Substituting these values into the formula, we get:
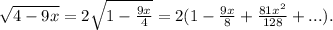
As we're considering |x|, we take
 terms, which gives us
terms, which gives us
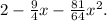
Now, to approximate √(310) using this expansion, we set (4-9x = 310) and solve for x. Rearranging gives us
 However, as we need |x|, we take
However, as we need |x|, we take
 . Substituting this value into the expansion gives us
. Substituting this value into the expansion gives us
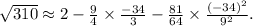
After the calculations, √(310) is approximately 17.605 when rounded to three decimal places.