Question

Special Right Triangles

Find the value of x.

(Round to the nearest tenth.)

Answer 1

x = 58

Explanation:

using the sine or cosine ratios in the right triangle and the exact values

• sin30° = cos60° =
`(1)/(2)` , then

sin 30° =
`(opposite)/(hypotenuse)` =
`(29)/(x)` =
`(1)/(2)` ( cross multiply )

x = 2 × 29 = 58

or

cos60° =
`(adjacent)/(hypotenuse)` =
`(29)/(x)` =
`(1)/(2)` ( cross multiply )

x = 2 × 29 = 58

Answer 2

`\sf x \approx \boxed{\; \; 58 \;\; }`

Explanation:

We can either Sine ratio or cosine ratio to find the value of x in right angled triangle.

If we use sine ratio

`\sf sin(30^\circ) = (opposite)/(hypotenuse )= (29)/(x)`

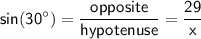
Solve for x.

`\sf x = (29)/(sin(30^\circ))`

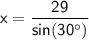
Substitute the value of sin(30°) = ½

`\sf x = (29)/((1)/(2))`

`\sf x = 29\cdot 2`

`\sf x = 58`

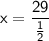
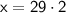
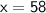
If we use cosine ratio

`\sf cos(60^\circ) = (adjacent)/(hypotenuse )= (29)/(x)`

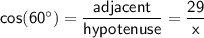
Solve for x.

`\sf x = (29)/(cos(60^\circ))`

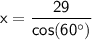
Substitute the value of cos(60°) = ½

`\sf x = (29)/((1)/(2))`

`\sf x = 29\cdot 2`

`\sf x = 58`

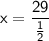
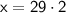
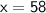
So, the value of x is 58.