Question

select all the points of intersection between the graphs of the function a(x)=(x+4)(x-1) and b(x)=(-3x+1)(x-1)

Answer 1

The graphs intersect at two points:

x = 1

x = -3/4

Let's find all the points of intersection between the graphs of the functions a(x)=(x+4)(x-1) and b(x)=(-3x+1)(x-1).

We can find these points by solving the system of equations formed by setting the two functions equal to each other:

a(x)=b(x)

Steps to solve:

1. Distribute both sides:

(x+4)(x−1)=(−3x+1)(x−1)

`x^(2) -x+4(x-1)=-3x(x-1)+1(x-1)`

`x^(2) -x+4x-4=-3x^(2) +3x+x-1`

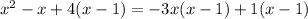
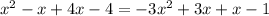
2. Combine like terms:

`x^(2) +3x-4=-3x^(2) 2+4x-1`

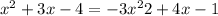
3. Move terms to one side and combine like terms:

`x^(2) +3x-4-(-3x^(2) +4x-1)=0`

`4x^(2) +3x-3=0`

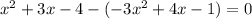
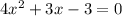
4. Solve the quadratic equation:

`x= \frac{-b+\sqrt{b^(2)-4ac } }{2a}`

`x= \frac{-3+\sqrt{3^(2)-4(4)(-3) } }{2(4)}`

`x=(1+7)/(8)`

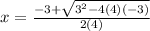
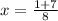
5. Separate the solutions:

x=1

`x=-(3)/(4)`