The solution to the equation
 is
is
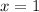 .
.
To solve the equation
 for
for
 , we can follow these steps:
, we can follow these steps:
1. Combine logarithmic terms:
![\[ \ln(x^3) + \ln(3^5) = (3)/(x) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/guyc52xadje12h7qggrllh3v9ea98sycly.png)
2. Combine logarithmic terms further:
![\[ \ln\left(3^5 \cdot x^3\right) = (3)/(x) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/fs9wvfjiota1viaarg1jae50i65r5fmo89.png)
3. Set the expression equal to
 :
:
![\[ 3x = (3)/(x) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ntd48r0dct88j8amnjcvdq19w8d43mft88.png)
Now, solve for
 :
:
![\[ 3x^2 = 3 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/gap4r6ao6ogpo552dhf6qagiicqny2qhdo.png)
Divide both sides by 3:
![\[ x^2 = 1 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/6qf8z1q0e5utiqgu6c99py39pfgm365g06.png)
Take the square root of both sides:
![\[ x = \pm 1 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/6lwjqoc9fdoi9wl0q4pjrgymvfqb2duvwr.png)
However, since the natural logarithm is only defined for positive values, we discard the negative solution.
Therefore, the solution to the equation
 is
is
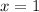 .
.