Answer:
$46.33
Explanation:
To calculate the future value of money considering inflation, we can use the formula for compound interest in reverse. In this case, it's a decrease due to inflation, so we use the formula:
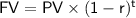
Where:
 is the future value (what $100 will buy we in the future),
is the future value (what $100 will buy we in the future),-
 is the present value (initial amount of money, $100 in this case),
is the present value (initial amount of money, $100 in this case),
 is the rate of decrease due to inflation per year (5%, or 0.05 in decimal form), and
is the rate of decrease due to inflation per year (5%, or 0.05 in decimal form), and-
 is the number of years (15 years in this case).
is the number of years (15 years in this case).
Now, substitute the values:

Calculate this expression to find the future value:
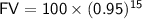
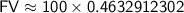
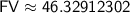

Therefore, 100 will buy approximately $46.33 worth of goods in 15 years, considering a 5% annual decrease in the value of money due to inflation.