Answer: x= 4.5, y=3.6, z=6.5
Explanation:
To find : x, y and z.
First label the triangle as A,B,C and D (as in the picture below).
ΔABC , ΔACD and ΔABD both are right triangles.
In ΔABC ,
 [By Pythagoras theorem]
[By Pythagoras theorem]
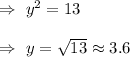
In ΔACD ,
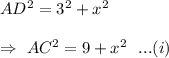 [By Pythagoras theorem]
[By Pythagoras theorem]
In ΔABC ,
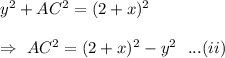
From (i) and (ii), we get

Z = 2+x= 2+4.5 =6.5
Hence, x= 4.5, y=3.6, z=6.5