The initial concentration of the reaction was approximately 73.21 M. The half-life of the reaction is approximately 17.72 seconds.
The initial concentration of the reaction can be determined using the first-order reaction equation:
ln(Cf / Ci) = -kt
where Ci is the initial concentration, Cf is the final concentration, k is the rate constant, and t is the time.
In this case, Cf = 0.256 M, Ci is what we want to find, k = 0.039/second, and t = 145 seconds.
Rearranging the equation, we have:
ln(Cf / Ci) = -kt
ln(0.256 / Ci) = -0.039 * 145
Using logarithmic properties, we can rewrite the equation as:
ln(Ci / 0.256) = 0.039 * 145
Taking the exponential of both sides, we have:
Ci / 0.256 =
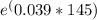
Now, solve for Ci:
Ci = 0.256 *
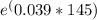
Ci ≈ 0.256 *

Ci ≈ 0.256 * 285.72
Ci ≈ 73.21 M
Therefore, the initial concentration of the reaction was approximately 73.21 M.
To find the half-life, we can use the equation:
t1/2 = (ln2) / k
where t1/2 is the half-life and k is the rate constant.
Plugging in the given value of k:
t1/2 = (ln2) / 0.039
t1/2 ≈ 17.72 seconds
Therefore, the half-life of the reaction is approximately 17.72 seconds.