The value of sinθ is -√13/4.
To find the value of sinθ, we can use the identity
 θ +
θ +
 θ = 1. Given that cos(-θ) = √3/4 and sinθ < 0, we can solve for sinθ as follows:
θ = 1. Given that cos(-θ) = √3/4 and sinθ < 0, we can solve for sinθ as follows:
1. Recall that cos(-θ) is equal to cosθ. So, we have cosθ = √3/4.
2. Using the identity
 θ +
θ +
 θ = 1, we can substitute the value of cosθ to solve for sinθ. This gives us:
θ = 1, we can substitute the value of cosθ to solve for sinθ. This gives us:
 θ +
θ +
 = 1
= 1
 θ + 3/16 = 1
θ + 3/16 = 1
 θ = 1 - 3/16
θ = 1 - 3/16
 θ = 13/16
θ = 13/16
3. Since sinθ < 0, we know that sinθ is negative.
4. Taking the square root of both sides, we find:
sinθ = -
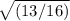
Simplifying further, sinθ = -√13/4.
Therefore, the value of sinθ is -√13/4.