Question

The radioisotope technetium-99 is often used as a radiotracer to detect disorders of the body. It has a half-life of 6.00 hours. If a patient received a 20.0 mg dose of this isotope during a medical procedure, how much would remain 24.0 hours after the dose was given?

Answer 1

After 24.0 hours, which equals four half-lives of the radioisotope technetium-99 with a half-life of 6.00 hours, only 1.25 mg of the original 20.0 mg dose would remain.

Step-by-step explanation:

The question is related to the decay of the radioisotope technetium-99 used as a radiotracer. Given that technetium-99 has a half-life of 6.00 hours, we can calculate the remaining amount of a 20.0 mg dose after 24.0 hours (which equals 4 half-lives) using the formula for exponential decay.

To find out how much of the radioisotope remains after a certain number of half-lives, we use the formula:

Remaining amount = Initial amount ×
`1/2^(number of half-live)`

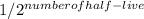
For technetium-99 after 24 hours:

• Initial amount = 20.0 mg
• Number of half-lives = 24 hours / 6 hours per half-life = 4
• Remaining amount = 20.0 mg × (1/2)4 = 20.0 mg × 1/16
• Remaining amount = 1.25 mg

Therefore, after 24.0 hours, 1.25 mg of the original 20.0 mg dose of technetium-99 would remain in the patient's body.

Answer 2

`0.25\ \text{mg}`

Step-by-step explanation:

`t_(1/2)` = Half-life of technetium-99 = 6 hours

`N_0` = Initial mass of sample = 20 mg

`t` = Time elapsed = 24 hours

Amount of mass remaining is given by

`N=N_0e^{-(\ln 2)/(t_(1/2))t}\\\Rightarrow N=20e^{-(\ln 2)/(6)* 24}\\\Rightarrow N=0.25\ \text{mg}`

The amount of the sample that would remain is
`0.25\ \text{mg}`.