Question

8x+≥-21
Solve the inequality pls give me the step by step of how you did it

Answer 1

The solution is
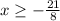`\( x \geq -(21)/(8) \)` or in interval notation
`\([- (21)/(8), \infty)\).`

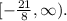
To solve the inequality
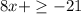`\(8x + \geq -21\)`, we'll follow these steps:

1. Subtract 8x from both sides:

`\[ 8x + 8x + \geq -21 - 8x \]`

This simplifies to:

`\[ 0 \geq -21 - 8x \]`

2. Add 21 to both sides to isolate the term with x:

`\[ 0 + 21 \geq -21 - 8x + 21 \]`

This simplifies to:

`\[ 21 \geq -8x \]`

3. Divide both sides by -8 (note: since we're dividing by a negative number, the inequality sign flips):

`\[ (21)/(-8) \leq (-8x)/(-8) \]`

This results in:

`\[ -(21)/(8) \leq x \]`

So, the solution to the inequality is x such that x is greater than or equal to
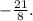`\(-(21)/(8)\).` In interval notation, the solution is
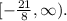`\([- (21)/(8), \infty)\).` This means any value of x within or greater than this interval satisfies the original inequality.