Final answer:
To convert from polar to rectangular form, the coordinates are determined by multiplying the polar radius by the cosine and sine of the angle respectively. For 4(cos(120°) + isin(120°)), the conversion results in the rectangular form of -2 + 2√3i.
Step-by-step explanation:
To convert
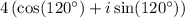 into rectangular form, we use Euler's formula,
into rectangular form, we use Euler's formula,
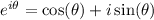 . Here,
. Here,
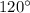 is our angle, so we have
is our angle, so we have
 .
.
Step 1: Evaluate the trigonometric functions at
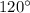 :
:
![\[ \cos(120^\circ) = -(1)/(2) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/7i92yqmb6wtxkhhu95b0y7ty9low8hbtng.png)
![\[ \sin(120^\circ) = (√(3))/(2) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/g0ilawhg6tqeb27iw34mt7h0zmyhowj3px.png)
Step 2: Substitute these values into Euler's formula:
![\[4 \left( -(1)/(2) + i(√(3))/(2) \right)\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/gi2ytfyqhv3gde0mkstuvpf3qc8s3woint.png)
This gives us the rectangular form of the complex number. For 4(cos(120°) + isin(120°)), the conversion results in the rectangular form of -2 + 2√3i.
Euler's formula is a powerful tool in complex number representation, enabling the conversion between polar and rectangular forms. Understanding this formula is fundamental in various mathematical and engineering applications, including signal processing and control systems.