Question

4 Consider the triangle below.

Part A: If AAMG is an isosceles triangle with base AG, what is the value of x?
Justify your answer.

Part B: What is the length of each leg?

Part C: What is the length of the base?

Answer 1

=>> Solution (part A) :

Given :

▪︎Triangle AMG is an isosceles triangle.

▪︎Measure of segment AM = (x+1.4) inches

▪︎Measure of segment MG = (2x+0.1) inches

▪︎Measure of segment AG = (3x-0.4) inches

▪︎segment AG is the base of triangle AMG.

Since AG is the base of the isosceles triangle AMG, segment AM and segment MG will be equal.

Which means :

`= \tt x + 1.4 = 2x + 0.1`

`= \tt x + 1.4 - 0.1 = 2x`

`= \tt \: x + 1.3 = 2x`

`= \tt 1.3 = 2x - x`

`\color{plum} \hookrightarrow \tt x = 1.3`

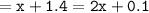
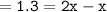
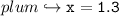
Thus, the value of x = 1.3

Therefore :

▪︎The value of x = 1.3

=>> Solution (Part B) :

We know that :

▪︎The value of x = 1.3

Which means :

The length of the leg AM :

`= \tt x + 1.4`

`= \tt 1.3 + 1.4`

`\color{plum} \tt leg \: AM= 2.7 \: inches`

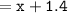
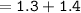
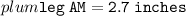
Thus, the length of the leg AM = 2.7 inches

The length of leg MG :

`= \tt 2x + 0.1`

`= \tt2 * 1.3 + 0.1`

`= \tt 2.6 + 0.1`

`\color{plum} \tt\: leg \:MG = 2.7 \: inches`

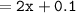
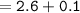
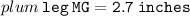
Thus, the length of the leg MG = 2.7 inches

Since the measure of the two legs are equal (2.7=2.7), we can conclude that we have found out the correct length of each leg.

Therefore :

▪︎Measure of leg AM = 2.7 inches

▪︎Measure of leg MG = 2.7 inches

=>> Solution (part C) :

We know that :

Value of x = 1.3

Then, measure of the base AG :

`= \tt 3x - 0.4`

`= \tt 3 * 1.3 - 0.4`

`= \tt 3.9 - 0.4`

`\color{plum}\tt \: Base \: AG = 3.5 \: inches`

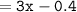
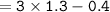
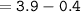
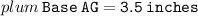
Thus, the measure of the base = 3.5 inches

Therefore :

▪︎ the length of base AG = 3.5 inches.