Question

Consider the equation y'+3y=te−2ty′+3y=te-2t

State the integrating factor μ(t)μ(t) and find the general solution y(t)y(t). Use c for the constant in your answer.

μ(t)μ(t)=

y(t)y(t)=

Answer 1

The integrating factor is μ(t) =
`e^(^3^t^)`, and the general solution to the differential equation y' + 3y =
`te^(^-^2^t^)` is given by y(t) = c *
`e^(^-^3^t^)` + (1/3) * t *
`e^(^-^2^t^)`, where c is the constant of integration.

Step-by-step explanation:

To solve the given first-order linear differential equation y' + 3y =
`te^(^-^2^t^),` we can use an integrating factor μ(t). The integrating factor is calculated using the coefficient of y, which is 3 in this case. The integrating factor (μ(t)) is given by the formula μ(t) =
`e^(^∫^3^d^t^) = e^(^3^t^)`.

Now, multiply both sides of the differential equation by the integrating factor μ(t):

`e^(^3^t^) * (y' + 3y) = e^(^3^t^) * (te^(^-^2^t^))`

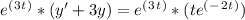
This simplifies to d/dt (e^(3t) * y) = te^(t). Integrate both sides with respect to t:

`∫d/dt (e^(^3^t^) * y) dt = ∫te^(^t^) dt`

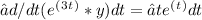
The left side integrates to
`e^(^3^t^)` * y, and the right side integrates to (1/3) * t *
`e^(^-^2^t^)` + c, where c is the constant of integration. Therefore,
`e^(^3^t^)` * y = (1/3) * t *
`e^(^-^2^t^)` + c.

Solving for y, we get y(t) = c *
`e^(^-^3^t^)` + (1/3) * t *
`e^(^-^2^t^),` which is the general solution to the given differential equation. The constant c is determined by the initial conditions if provided. This solution describes the family of curves that satisfy the given differential equation.