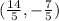Answer:
Known two points, list function expression, use


The function expression is
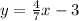
It is known that the cross section of two points is 7, so the point of 2:3 is 14 / 5, that is, 0 + 14 / 5 is the abscissa of the point, which is substituted into the original equation to get y = - 7 / 5.
So the result is