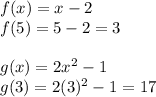Question

Functions f, g, and h are defined as follows: f(x) = x -2, g(x) = 2x^(2)-1, and h(x) =2x^(3)+4.

Find the inverse of function g(x).
(b) Find f(h(-2)).
(c) Find g(f(5))

Answer 1

a) g^-1(x) = - √2x+1, √2x+1

2 2

b) -14

c) 17

Explanation:

a) g(x) = 2x² - 1

y= 2x² - 1 becomes x = 2y² - 1

Solve for y: x = 2y² - 1

g^-1(x) = - √2x+1, √2x+1

2 2

*answer is: -√2x+1 (over) 2 & √2x+1 (over) 2*

b) Find: f(h(-2))

h(x) = 2x³ +4

h(-2) = 2(-2)³ + 4

= - 12

f(x) = x – 2

f(-12) = (-12) – 2

= -14

c) Find: g(f(5))

f(x) = x – 2

f(5) = (5) – 2

= 3

g(x) = 2x² - 1

g(3) = 2(3)² - 1

= 17

Answer 2

(a.): There is no inverse of g(x). 2x^(2)-1 is not a one-to-one function, meaning there is not one x for every y (on a graph, you can apply the horizontal line test and see that the graph crosses the same x-coordinate for many y-coordinates twice). Thus, it does not have an inverse

(b.): f(h(-2)) = -14

(c.): g(f(5)) = 17

Explanation:

(a.): g(x) = 2x^(2) - 1 is not a one-to-one function, meaning there is not one x for every y (on a graph, you can apply the horizontal line test and see that the graph crosses the same x-coordinate for many y-coordinates twice). Thus, it does not have an inverse.

(b.) To find f(h(-2)), we first plug in -2 as the input for h(x). Then, this output becomes the input for f(x):

`h(x)=2x^3+4\\h(-2)=2(-2)^3+4=-12\\\\\f(x)=x-2\\f(-12)=-12-2=-14`

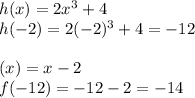
(c.) To find g(f(5)) we first plug in 5 as the input for f(x). Then, this output becomes the input for g(x):

`f(x)=x-2\\f(5)=5-2=3\\\\g(x)=2x^2-1\\g(3)=2(3)^2-1=17`