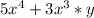To simplify the expression
![[tex]3x ^ 3 * (2x + y)](https://img.qammunity.org/2024/formulas/mathematics/high-school/odudjnh6r3srgkhpuzsn8qf1rvkscoe3o7.png) [/tex], we can distribute the
[/tex], we can distribute the
![[tex]3x ^ 3](https://img.qammunity.org/2024/formulas/mathematics/high-school/jlybrcgra5rbx0wmxfvkyufgr0w0hwbwqy.png) [/tex] to both terms inside the parentheses. The equivalent expression is
[/tex] to both terms inside the parentheses. The equivalent expression is
![[tex]6x^4 + 3x ^ 3 * y](https://img.qammunity.org/2024/formulas/mathematics/high-school/rxjamq7o98gkerj5jhx6vm2b674frbpvm4.png) [/tex].
[/tex].
The given expression is
![[tex]3x ^ 3 * (2x + y)](https://img.qammunity.org/2024/formulas/mathematics/high-school/odudjnh6r3srgkhpuzsn8qf1rvkscoe3o7.png) [/tex].
[/tex].
To simplify this expression, we can use the distributive property of multiplication over addition.
We multiply
![[tex]3x ^ 3](https://img.qammunity.org/2024/formulas/mathematics/high-school/jlybrcgra5rbx0wmxfvkyufgr0w0hwbwqy.png) [/tex] with both terms inside the parentheses:
[/tex] with both terms inside the parentheses:
![[tex]3x ^ 3 * 2x + 3x ^ 3 * y](https://img.qammunity.org/2024/formulas/mathematics/high-school/l61m0hdaq0cfy8pjcf5et9t594zq3bfcji.png) [/tex]
[/tex]
Applying the power rule of exponents,
![[tex]x^n * x^m = x^(n+m)](https://img.qammunity.org/2024/formulas/mathematics/high-school/krod47g5doid52zn7t9p49s963jszh6xio.png) [/tex], the first term simplifies to
[/tex], the first term simplifies to
![[tex]6x^4](https://img.qammunity.org/2024/formulas/mathematics/high-school/axp1vji0qmenaseew59jgti6x0iu7u0cfm.png) [/tex].
[/tex].
The second term remains as it is,
![[tex]3x ^ 3 * y](https://img.qammunity.org/2024/formulas/mathematics/high-school/u7eejl54hv9jnbal81lpveh92acrmq6rgt.png) [/tex].
[/tex].
Therefore, the equivalent expression is
![[tex]6x^4 + 3x ^ 3 * y](https://img.qammunity.org/2024/formulas/mathematics/high-school/rxjamq7o98gkerj5jhx6vm2b674frbpvm4.png) [/tex].
[/tex].
Question:
Which is equivalent to
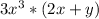
A.

B.
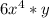
C.
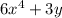
D.
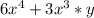
E.