The 90% confidence interval for the mean is 12.6 to 13.2 reproductions per hour.
We are interested in constructing a 90% confidence interval for the mean number of virus reproductions. We know the population standard deviation is σ=2.2 reproductions per hour, and we have a sample size of n=358.
The margin of error is found with the formula E=
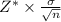
The confidence level is 90%, so we find the corresponding z-score using the normalcdf function on most calculators. The output is 1.645.
The margin of error is then
E = 1.645⋅
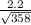
E = 0.30 reproductions per hour.
The mean number of reproductions per hour is μ=12.9, so the 90% confidence interval is μ ± E = 12.9 ± 0.30 = (12.60,13.20) reproductions per hour.