Question

Is this the graph of 9(x) = (x - 1)^2 (x + 2) or

h(x) = (x - 1) (x + 2)^2? Explain how you know.

Answer 1

The polynomial shown in the graph is the first option:

g(x) = (x - 1)²*(x +2)

Which function is shown in the graph?

Ok, for a polynomial, if the graph just passes through the x-axes like in the left intercept, then the multiplicity of that zero is odd.

If it passes through the x-axis like in the right intercept (so we have like the vertex of a parabola) then we have an even multiplicity.

Remember that if a polynomial has zeros x = h, and x = k, then the equation:

`y = (x - h)^n*(x - k)^m`

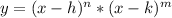
Means that the multiplicity of h is n, and the multiplicity of k is m.

From this, we can see that:

• The zero at x = -2, must have odd multiplicity.
• The zero at x = 1, must have even multiplicity.

Then the correct option is:

g(x) = (x - 1)²*(x +2)