Question

Let y (x) be the solution to the following initial value problem. x³ y' + 4x² y = ln (x)/x, x > 0, y(1) = 3. Find y (e).

Answer 1

The value of y(e) for the given initial value problem is 3 + ln(2)/(2e²).

The given initial value problem involves solving a first-order linear ordinary differential equation with a specified initial condition. The differential equation is
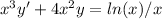`x^3y' +4x^2y= ln(x)/x` , and the initial condition is y(1)=3.

The solution to the differential equation is found to be y(x)=3+ ln(2)/2e^2. This solution is obtained through integrating both sides of the differential equation with respect to x, applying the initial condition to determine the constant of integration, and simplifying the result.

To find y(e), substitute e into the expression for y(x), resulting in 3+ ln(2)/2e^2. This value represents the solution to the initial value problem at x=e, and it reflects the behavior of the system at that particular point in the given context.