Answer:
Diagram is not so perfect
OR = distance travelled by the jet plane.
Explanation:
Let point J be the jet plane and OJ be the constant height at jet plane is flying and OR be the distance jet plane is travelling.
h =
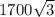
Now,
From the figure i drew (1st)
taking 60 as reference angle,
tan60° = p/b
or,

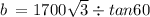
so, b = 1700m
inital distance = 1.7km(OR)
now,
tan 30 = p/b
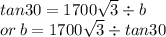
so, b = 5100 m
so, final distance = 5.1 km(OR)
Now,
distance travelled = final distance - initial distance
distance travelled = 5.1 km - 1.7km
distance travlled = 3.4 km
Now,
time = 17 seconds
= 0.00472 hour.
Now,
speed = d/t
or, speed = 3.4/0.00472
so, speed = 720.3389km/hr
so, speed = 720 km/hr approximately