Question

Points of the curve x²+2y²=1 that extremum f(x,y)=xy

Answer 1

The points on the curve
`\(x^2 + 2y^2 = 1\)` where the function f(x, y) = xy has extremum are:

1.
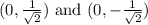
`\((0, (1)/(√(2)))\) and \((0, -(1)/(√(2)))\)`

2.
`\(((1)/(2), (1)/(2√(2)))\) and \((-(1)/(2), -(1)/(2√(2)))\)`

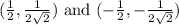
3.
`\(((1)/(2), -(1)/(2√(2)))\) and \((-(1)/(2), (1)/(2√(2)))\)`

To find the points on the curve
`\(x^2 + 2y^2 = 1\)` where the function f(x, y) = xy has extremum, we can use the method of Lagrange multipliers. The extremum points occur when the gradient of the function f is parallel to the gradient of the constraint
`\(x^2 + 2y^2 = 1\)`.

Let's define the Lagrangian function:

`\[ L(x, y, \lambda) = xy + \lambda(1 - x^2 - 2y^2) \]`

Now, we need to find the partial derivatives of L with respect to
`\(x\), \(y\), and \(\lambda\)`, and set them equal to zero:

1.
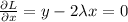
`\((\partial L)/(\partial x) = y - 2\lambda x = 0\)`

2.
`\((\partial L)/(\partial y) = x - 4\lambda y = 0\)`

3.
`\((\partial L)/(\partial \lambda) = 1 - x^2 - 2y^2 = 0\)`

Solving this system of equations will give us the critical points.

Let's solve the system:

From equation (1), we have
`\(y - 2\lambda x = 0 \implies y = 2\lambda x\)`

Substitute this into equation (2):
`\(x - 4\lambda y = 0 \implies x - 4\lambda(2\lambda x) = 0\)`

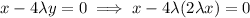
Simplify:
`\(x - 8\lambda^2 x = x(1 - 8\lambda^2) = 0\)`

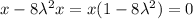
This gives two possibilities:

1. x = 0

2.
`\(1 - 8\lambda^2 = 0 \implies \lambda = \pm(1)/(√(8)) = \pm(1)/(2√(2))\)`

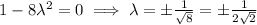
Now, we can use the third equation to find the corresponding values of y for each case.

For x = 0, we substitute into
`\(1 - x^2 - 2y^2 = 0\) to get \(1 - 2y^2 = 0\), which gives \(y = \pm(1)/(√(2))\).`

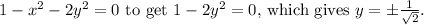
For
`\(\lambda = \pm(1)/(2√(2))\)`, substitute into
`\(1 - x^2 - 2y^2 = 0\)` to find the corresponding values of x and y.

Now, we have three sets of solutions for (x, y):

1.
`\( (0, (1)/(√(2))) \) and \( (0, -(1)/(√(2))) \)`

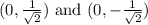
2. For
`\(\lambda = (1)/(2√(2))\):`

-
`\( ((1)/(2), (1)/(2√(2))) \) and \( (-(1)/(2), -(1)/(2√(2))) \)`

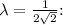
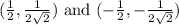
3. For
`\(\lambda = -(1)/(2√(2))\):`

-
`\( ((1)/(2), -(1)/(2√(2))) \) and \( (-(1)/(2), (1)/(2√(2))) \)`

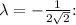
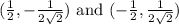
These are the points on the curve where the function f(x, y) = xy has extremum.

The probable question may be:

Find points of the curve x²+2y²=1 having extremum f(x,y)=xy