Question

Clayton wants to be a musician. After school one afternoon, he spends ___ of his time practicing the drums and 3/8 of the remaining time working on homework and eating dinner. He spends the remaining ___ texting and talking to his friends. How long did he practice the drums?

Answer 1

Clayton spends
`\( \underline{(5)/(8)} \)` of his time practicing the drums, and
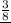`\( \underline{(3)/(8)} \)` of the remaining time on homework and dinner. The remaining
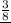`\( \underline{(3)/(8)} \)` is spent texting and talking to friends.

Step-by-step explanation:

Let ( T ) represent the total time Clayton has after school. He spends
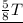`\( \underline{(5)/(8)T} \)` practicing the drums. The remaining time is
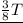`\( \underline{(3)/(8)T} \)`. Now,
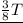`\( \underline{(3)/(8)T} \)` is split between homework and dinner, leaving
`\( \underline{(5)/(8)} \)` for practicing:

`\[ \underline{(3)/(8)T} = (3)/(8) * \underline{(3)/(8)T} + (3)/(8) * \underline{(5)/(8)T} \]`

Solving for
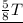`\( \underline{(5)/(8)T} \)` (time spent practicing), we get:

`\[ \underline{(5)/(8)T} = (9)/(64)T + (15)/(64)T \]`

Combining the terms on the right side:

`\[ \underline{(5)/(8)T} = (24)/(64)T \]`

Simplifying the fraction:

`\[ \underline{(5)/(8)T} = (3)/(4)T \]`

Thus, Clayton spends
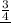`\( \underline{(3)/(4)} \)` of his total time practicing the drums.

In conclusion, Clayton spends
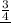`\( \underline{(3)/(4)} \)` of his time practicing the drums, which corresponds to
`\( \underline{(5)/(8)} \)` of his total time after school. This allocation of time aligns with his musical aspirations and ensures a balanced engagement in other activities.