Question

Complete the inequality so that it will be true for any value of x. -x^2 - 4x - 4...0

Option 1: >
Option 2: <
Option 3: =

Answer 1

The correct answer to complete the inequality

`-x^2 - 4x - 4 ... 0`

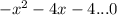
is '<' as the expression will always be less than zero for any real value of x.

Step-by-step explanation:

To complete the inequality

`-x^2 - 4x - 4 ... 0,`

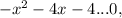
we need to determine the correct relational operator. Since the leading coefficient of the quadratic term

`(-x^2)`

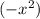
is negative, the parabola opens downward, which means it will always be less than a certain value depending on x. Therefore, for any value of x, the expression

`-x^2 - 4x - 4`

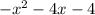
will always be less than zero. Thus, the correct answer to complete the inequality is < (Option 2).

Additionally, without specifically completing the square as per the provided context, we understand that the quadratic expression does not factor into a perfect square, and will not have a maximum value at zero for any real value of x. Hence, for all values of

`x, -x^2 - 4x - 4 < 0`

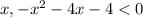
is the true statement.