Answer:
Isosceles triangle
Explanation:
A triangle is a polygon with three sides and three angles. Types of triangles are isosceles, equilateral, scalene and equilateral.
An isosceles triangle is a triangle with two equal sides and two equal angles.
The distance between two points
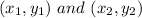 on the coordinate plane is given by:
on the coordinate plane is given by:
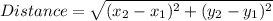
Given a triangle with vertices A(-1,-4), B(2,-4) and C(2,-1). The sides of the triangle is given by:
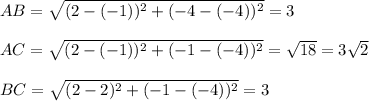
Since AB = BC, we can say that the triangle is an isosceles triangle.