Question

Differentiate y=x e^-x⁵
yʹ=

Answer 1

To differentiate the function y = x
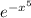`e^(-x^5)` with respect to x, we'll use the product rule and chain rule. The derivative of y = x
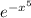`e^(-x^5)` with respect to x is
`\(e^(-x^5)` - 5x⁵
`e^(-x^5)\)`.

Step-by-step explanation:

To differentiate the function y = x
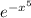`e^(-x^5)` with respect to x, we'll use the product rule and chain rule.

The product rule states that if u and v are functions of x, then the derivative of uv with respect to x is given by (uv)' = u'v + uv'.

Let u = x and v =
`e^(-x^5)\)`. Then, we have:

y = u v

Now, apply the product rule:

y' = u'v + uv'

The derivative of u with respect to x is 1, and the derivative of v with respect to x involves the chain rule:

v' = -5x⁴
`e^(-x^5)`

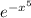
Now substitute these into the product rule formula:

y' = 1 ·
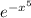`e^(-x^5)` + x · (-5x⁴
`e^(-x^5)`)

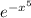Simplify:

y' =
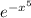`e^(-x^5)` - 5x⁵
`e^(-x^5)`

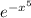
Therefore, the derivative of y = x
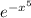`e^(-x^5)` with respect to x is
`\(e^(-x^5)` - 5x⁵
`e^(-x^5)\)`.