We've simplified each expression, we can clearly see that:
Expression 1:

Expression 2:

Expression 3:
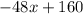
All three expressions are equivalent to one another, since each simplifies to the same combination of terms.
Therefore, the answer is option: A) Yes, the expressions are equivalent.
Let's examine each expression step by step to see if they are equivalent to the given expression
 .
.
**Expression 1:
 **
**
Simplify by distributing the 8 across each term inside the parentheses:
![\[8 \cdot 9 - 8 \cdot 6x + 8 \cdot 11 = 72 - 48x + 88\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/jgg9pv75jkgj85jlrng5mjoy6bcixfakpq.png)
Combine the constant terms:
![\[72 + 88 = 160\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/zfqf81vkb033j15fvizagsde7uhqbco3i6.png)
Now, the expression is:
![\[160 - 48x\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/awltsr5av194w6fpgulxi67tz41745rbb4.png)
**Expression 2:
 **
**
Before distributing, simplify the constant terms outside the parentheses:
![\[15 - 35 = -20\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/xrx223tjnusjgglf2ynj7uuj129y6ibnze.png)
Now, distribute
 across each term inside the parentheses:
across each term inside the parentheses:
![\[(3)/(2) \cdot (-32x) + (3)/(2) \cdot 120 = -48x + 180\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/qt2erj0ao44y17snmi4z9krsgov2lchonz.png)
Now, the expression is:
![\[180 - 48x - 20\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/qy7vskhvl519sicej39s5w33ha3yure3sj.png)
Combine the constant terms:
![\[180 - 20 = 160\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/67tulow7zgsir897u1tinvr2l9egdj0jz5.png)
So, the second expression becomes:
![\[160 - 48x\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/awltsr5av194w6fpgulxi67tz41745rbb4.png)
**Expression 3:
 **
**
Distribute the
 across each term inside the parentheses:
across each term inside the parentheses:
![\[-16 \cdot 3x + -16 \cdot (-10) = -48x + 160\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/81tq10kdp31mwnycgtlmlo1a6oh4hm81r6.png)
So, the third expression is:
![\[-48x + 160\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/8v6dfzbss8gtqehdrncgw0akjoui8xuukx.png)
Now that we've simplified each expression, we can clearly see that:
Expression 1:

Expression 2:

Expression 3:
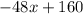
All three expressions are equivalent to one another, since each simplifies to the same combination of terms.
Therefore, the answer is:z
A) Yes, the expressions are equivalent.