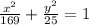Answer:
The equation of the elipse is
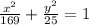
Explanation:
Equation of an elipse:
The equation of a elipse with centre
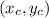 has the following format:
has the following format:
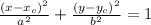
Centre at (0,0):
Means that
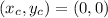 .
.
So
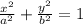
Vertex at (13,0):
Vertex at 13 means that
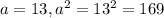
Focus at (-12,0):
Means that
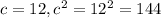
We have that:
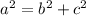
So
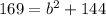
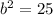
So, the equation of the elipse is