Final Answer:
The value of ( m ) is 3.The expression
 Setting this equal to zero gives m = 3 ), confirming the value that satisfies the condition for mx - 1 to be a factor.
Setting this equal to zero gives m = 3 ), confirming the value that satisfies the condition for mx - 1 to be a factor.
Step-by-step explanation:
To find the value of m , we can use the fact that mx - 1 is a factor of the given polynomial. This means that if we substitute
 into the polynomial, the result should be zero.
into the polynomial, the result should be zero.
The given polynomial is8x⁴ + 4x ³ - 16x + 10x + m. Substituting \
 , we get
, we get
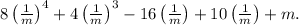
Now, let's simplify each term:
1.
 (since any number to the power of 4 is still that number).
(since any number to the power of 4 is still that number).
2.
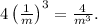
3.
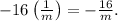
4.
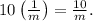
5. m remains unchanged.
Now, add all these terms:
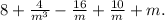
To make this expression equal to zero, the coefficient of each term must be zero. Therefore, we set
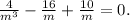
Solving this equation, we find m = 3 which is the final answer.