Question

In the United States, approximately 74% of owned dogs are spayed or neutered. If 31 owned dogs are randomly selected, calculate the probability that exactly 24 of them are spayed or neutered.

Answer 1

To calculate the probability that exactly 24 out of 31 randomly selected owned dogs are spayed or neutered in the United States, use the binomial probability formula.

Step-by-step explanation:

To calculate the probability that exactly 24 out of 31 randomly selected owned dogs are spayed or neutered in the United States, we need to use the binomial probability formula. The formula is:

`P(x) = C(n, x) * p^x * (1-p)^(^n^-^x^)`

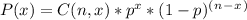
Where:
n = number of trials = 31
x = number of successes = 24
p = probability of success = 0.74
C(n, x) = combination of choosing x successes from n trials

Plugging in the values, we get:

`P(24) = C(31, 24) * 0.74^2^4 * 0.26^7`

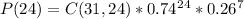
Using a calculator or software, we can calculate P(24) to find the probability that exactly 24 owned dogs are spayed or neutered in a random sample of 31 dogs in the United States.