Question

Endpoints of a diameter are P(-1,3) and Q(5,-1) . find length of diameter .

Answer 1

The length of the diameter between points P(-1,3) and Q(5,-1) is 10 units.

Step-by-step explanation:

To find the length of the diameter, we can use the distance formula in coordinate geometry. The distance formula between two points
`\((x_1, y_1)\) and \((x_2, y_2)\) is given by \(√((x_2 - x_1)^2 + (y_2 - y_1)^2)\).`

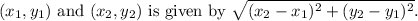
In this case, the coordinates of points P and Q are
`\((-1, 3)\) and \((5, -1)\)`respectively. Applying the distance formula:

`\[d = √((5 - (-1))^2 + (-1 - 3)^2)\]`

`\[d = √(6^2 + (-4)^2)\]`

`\[d = √(36 + 16)\]`

`\[d = √(52)\]`

`\[d = 2√(13)\]`

Hence, the length of the diameter, which is the distance between points P and Q, is
`\(2√(13)\)` units. To simplify further,
`\(√(52)\)` equals
`\(2√(13)\)`, which ultimately simplifies to
`\(10\)`units.