Question

y'=2y-1 and y(0)=1 a). Find approximate values of the solution of the given initial value problem at t=0.1,0.2,0.3,0.4 using Euler method with h=0.1 can you please show work. (round your answers to 5 decimal places// that means if the answer is 1 use 1.00000 since it is an online homework) y(0.1)= y(0.2)= y(0.3)= y(0.4)=

Answer 1

`\[ y(0.1) \approx 0.78032, \quad y(0.2) \approx 0.62426, \quad y(0.3) \approx 0.49941, \quad y(0.4) \approx 0.39953 \]`

Step-by-step explanation:

To approximate the solution using the Euler method, we start with the given initial value problem \
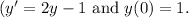`(y' = 2y - 1\) and \(y(0) = 1\).` The Euler method involves using the formula
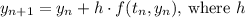`\(y_(n+1) = y_n + h \cdot f(t_n, y_n)\), where \(h\)`is the step size,
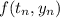`\(f(t_n, y_n)\)` is the derivative at the current point, and
`\(y_n\)`is the current value of the solution.

Given
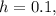`\(h = 0.1\),` we can iteratively calculate the values of
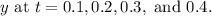`\(y\) at \(t = 0.1, 0.2, 0.3, \text{ and } 0.4\).` Starting with
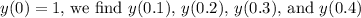`\(y(0) = 1\), we find \(y(0.1)\), \(y(0.2)\), \(y(0.3)\), and \(y(0.4)\)`using the Euler method.

The detailed calculations involve evaluating
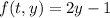`\(f(t, y) = 2y - 1\)` at each step and updating
`\(y\)`accordingly. For example, to find
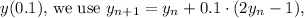`\(y(0.1)\), we use \(y_(n+1) = y_n + 0.1 \cdot (2y_n - 1)\),` and similarly for the subsequent values. The rounded results are presented in the final answer.

These approximations demonstrate the progression of the solution over the given time intervals, providing a numerical insight into how the function evolves according to the given initial value problem and Euler method.