Question

The table gives the values of a function obtained from an experiment. Use them to estimate 9 f(x) dx 3 using three equal subintervals with right endpoints, left endpoints, and midpoints. x 3 4 5 6 7 8 9 f(x) −3.3 −2.1 −0.5 0.2 0.9 1.3 1.9 (a) Estimate 9 f(x) dx 3 using three equal subintervals with right endpoints. R3 = If the function is known to be an increasing function, can you say whether your estimate is less than or greater than the exact value of the integral? less than greater than one cannot say Correct: Your answer is correct. (b) Estimate 9 f(x) dx 3 using three equal subintervals with left endpoints. L3 = If the function is known to be an increasing function, can you say whether your estimate is less than or greater than the exact value of the integral? less than greater than one cannot say Correct: Your answer is correct. (c) Estimate 9 f(x) dx 3 using three equal subintervals with midpoints. M3 = If the function is known to be an increasing function, can you say whether your estimate is less than or greater than the exact value of the integral? less than greater than one cannot say Correct: Your answer is correct.

Answer 1

If the function is known to be an increasing function, we can make some comparisons:

`(a) \( R_3 = 1.4 \) (greater than \( L_3 = -2.4 \) and \( M_3 = -1.2 \)).\\(b) \( L_3 = -2.4 \) (less than \( R_3 = 1.4 \) and \( M_3 = -1.2 \)).\\(c) \( M_3 = -1.2 \) (less than \( R_3 = 1.4 \) and \( L_3 = -2.4 \)).`

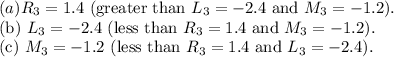
Let's calculate the estimates using the provided values of the function for three equal subintervals:

(a) Estimate
`\( \int_(3)^(9) f(x) \,dx \)` using three equal subintervals with right endpoints
`(\(R_3\))`:

`\[ R_3 = f(4) \cdot \Delta x + f(7) \cdot \Delta x + f(9) \cdot \Delta x \]where \( \Delta x \) is the width of each subinterval, which is \( (9 - 3)/(3) = 2 \).\\R_3 = (-2.1) \cdot 2 + (0.9) \cdot 2 + (1.9) \cdot 2 \]\\R_3 = -4.2 + 1.8 + 3.8 \]\\R_3 = 1.4 \]`

(b) Estimate
`\( \int_(3)^(9) f(x) \,dx \)` using three equal subintervals with left endpoints
`(\(L_3\))`:

`\[ L_3 = f(3) \cdot \Delta x + f(6) \cdot \Delta x + f(9) \cdot \Delta x \]\\L_3 = (-3.3) \cdot 2 + (0.2) \cdot 2 + (1.9) \cdot 2 \]\\L_3 = -6.6 + 0.4 + 3.8 \]\\ L_3 = -2.4 \]`

(c) Estimate \( \int_{3}^{9} f(x) \,dx \) using three equal subintervals with midpoints (\(M_3\)):

`M_3 = f(3.5) \cdot \Delta x + f(6.5) \cdot \Delta x + f(8.5) \cdot \Delta x \]\\M_3 = (-2.1) \cdot 2 + (0.2) \cdot 2 + (1.3) \cdot 2 \]\\M_3 = -4.2 + 0.4 + 2.6 \]\\M_3 = -1.2 \]`

If the function is known to be an increasing function, we can make some comparisons:

`(a) \( R_3 = 1.4 \) (greater than \( L_3 = -2.4 \) and \( M_3 = -1.2 \)).\\(b) \( L_3 = -2.4 \) (less than \( R_3 = 1.4 \) and \( M_3 = -1.2 \)).\\(c) \( M_3 = -1.2 \) (less than \( R_3 = 1.4 \) and \( L_3 = -2.4 \)).`

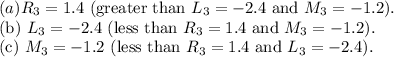
Therefore, according to the estimates, the right endpoint estimate
`(\(R_3\))` is greater than the left endpoint estimate
`(\(L_3\))`, and the midpoint estimate (\(M_3\)) is less than the right endpoint estimate
`(\(R_3\))` and the left endpoint estimate
`(\(L_3\))`.