Question

Find P(5) when p = 0.10.

Answer 1

P(5) = Px5. P=0.10, so 0.10x5= 0.5

0.5 is your answer.

Answer 2

The probability P(5) represents the chance of getting exactly 5 successes in a binomial distribution. With p = 0.10, in a single trial, this probability is 0 as it requires more trials for 5 successes.

In probability theory, P(5) usually represents the probability of getting exactly 5 successes in a fixed number of trials in a binomial distribution. Given p = 0.10, which is the probability of success on a single trial, we can use the binomial probability formula:

`\[ P(X = k) = \binom{n}{k} p^k (1-p)^(n-k) \]`

where n is the number of trials, k is the number of successes, p is the probability of success on a single trial, and (1-p) is the probability of failure on a single trial.

For P(5), assuming a single trial (n = 1), the formula simplifies to:

`\[ P(5) = \binom{1}{5} (0.10)^5 (0.90)^(1-5) \]`

However,
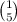`\( \binom{1}{5} \)` is 0 because you can't have 5 successes in a single trial. Therefore, P(5) when p = 0.10 is 0.