Answer:
5
Explanation:
You want me to let you in on a secret? There's a simple equation that you can use to solve any quadratic problem in a minute or two.
It's the quadratic formula
x =
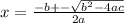
The +- means that you will do this two times, the first time you will do -b + sqrt(b^2-4ac), and the second time you will do minus that.
a is the coefficient of the first term in the expression, b is the second and c is the third.
so we get
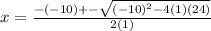 , which is the same as
, which is the same as
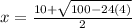
and
x =
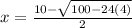
The top part simplifies to
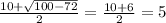 , which is your answer.
, which is your answer.