Question

Find the cube root of 9 using binomial expansion.

a. ∛9 = (1 + 2/3)^3
b. ∛9 = (1 - 2/3)^3
c. ∛9 = (1 + 3/2)^3
d. ∛9 = (1 - 3/2)^3

Answer 1

To find the cube root of 9 using binomial expansion, we can use the binomial theorem. The cube root of 9 using binomial expansion is ∛9 = (1 + x)³. The answer is not in the answer choices.

Step-by-step explanation:

To find the cube root of 9 using binomial expansion, we can use the binomial theorem. The binomial theorem states that for any real numbers a and b and any positive integer n, (a + b)ⁿ can be expanded as:

(a + b)ⁿ =
`\sum_(k=0)^(n) \binom{n}{k} a^(n-k)b^k`

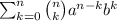
For the cube root of 9, we want to find (a + b)³ such that a³ = 9. We can choose a = 1 and b = x where x is a term that helps us achieve a³ = 9.

(1 + x)³ = 1³ +
`\binom{3}{1}1^2x` +
`\binom{3}{2}` 1x² + x³

Simplifying this expansion:

1 + 3x + 3x² + x³

Now, set this equal to 9:

1 + 3x + 3x² + x³ = 9

Solve for x:

x³ + 3x² + 3x - 8 = 0

It can be seen that x = 1 is a solution, so (1 + x)³ is a factor. Thus, (1 + x)³ = (1 - x)³ = 9.

Answer 2

The cube root of 9 can be approached using binomial expansion by expressing 9 as the cube of a number close to it, which would be 8 (2^3). We then express the cube root of 9 as (2 + 1/2)^3, which after binomial expansion and simplification approximates the cube root of 9.

Step-by-step explanation:

To find the cube root of 9 using binomial expansion, we should first express 9 as a cube of a number close to it, which would be the cube of 8 (23) or the cube of 27 (33). since 8 is closer to 9, we'll start with that. We can express 9 as 8 + 1, which is 23 + 1. the cube root of 9 can be re-written as the cube root of (23 + 1), which is equivalent to (2 + 1/2)3, because adding 1/2 to 2 gives us 2.53, which is 15.625, and this value is closer to 9 when we take the cube root.

To express this using binomial expansion:

• (2 + (1/2))3 = 23 + 3(22)(1/2) + 3(2)(1/2)2 + (1/2)3

After expansion, we round off and simplify as necessary to get an approximation of the cube root of 9. So option a is closer to the correct expression for the cube root of 9.