Answer:
BC ≈ 98.95 feet
Explanation:
From the figure attached,
A shark is swimming 42 feet below sea level at point A and a boat on the water is at point C.
If the angle of depression from the coat to shark is 23°,
tan(23°) =
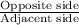
tan(23°) =
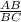
BC =
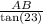
BC =
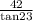
BC = 98.947
BC ≈ 98.95 feet