Answer:
The model is
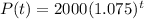 .
.
There will be 4122 fish in 10 years.
Explanation:
Exponentially increasing population:
An exponentially increasing population can be represented by the following model:
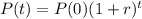
In which P(t) is the population after t years, P(0) is the initial population, and r is the growth rate, as a decimal.
A population of 2000 fish increases at an annual rate of 7.5%.
This means that

So
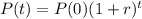
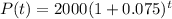
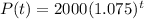
This is the model.
How many fish will there be in 10 years?
This is P(10).
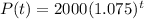

There will be 4122 fish in 10 years.