Question

Fill in the blanks. After conducting research around the country, Ford Motor Company determined that the probability of a randomly selected Ford vehicle on the road being driven by a female is 0.71. If there are 3,682 Ford vehicles in your town, around __________ cars will be driven by females, give or take __________. Assume each car represents an independent tria

Answer 1

2614 cars will be driven by females, give or take 28.

Explanation:

For each Ford vehicle, there are only two possible outcomes. Either it is driven by a female, or it is not. Cars are independent. So we use the binomial probability distribution to solve this question.

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

Can be approximated to a normal distribution, using the expected value and the standard deviation.

The expected value of the binomial distribution is:

`E(X) = np`

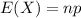
The standard deviation of the binomial distribution is:

`√(V(X)) = √(np(1-p))`

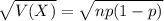
Company determined that the probability of a randomly selected Ford vehicle on the road being driven by a female is 0.71.

This means that
`p = 0.71`

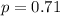
If there are 3,682 Ford vehicles in your town

This means that
`n = 3682`

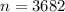
__________ cars will be driven by females, give or take __________.

The mean and the standard deviation complete this. So

Mean:
`E(X) = np = 3682*0.71 = 2614`

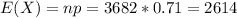
Standard deviation:
`√(V(X)) = √(np(1-p)) = √(3682*0.71*0.29) = 28`

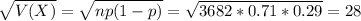
2614 cars will be driven by females, give or take 28.