Question

The force acting on a beam was measured 5 times under the same operating conditions. This process was repeated by 3 observers, resulting in 3 sets of data. The standard deviations of these data sets were: Standard Deviation 1= 1.11, Standard Deviation 2= 0.99, Standard Deviation 3= 1.15 The corresponding mean values were: 52.3, 51.5, and 52.7, respectively. Compute the Pooled Standard Deviation for these data sets. (Provide your answer using two decimal places)

Answer 1

The Pooled Standard Deviation for these data sets is 1.09

Step-by-step explanation:

Given that;

Standard Deviation 1 = 1.11

Standard Deviation 2 = 0.99

Standard Deviation 3 = 1.15

pooled standard deviation is given as;

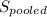`S_(pooled)` = (n1 - 1 )s1² + ..... + ( nk - 1 )sk² / n1 + ..... + nk - 1

where n1, n2....., nk are the sample sizes of k groups and s1, s2, ....., sk are the standard deviations of k groups respectively

so for the 3 groups;

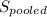`S_(pooled)`² = (n1 - 1 )s1² + (n2 - 1 )s2² + ( n3 - 1)s3² / n1 + n2 + n3 - 3

we substitute

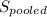`S_(pooled)`² = (5 - 1 )1.11² + (5 - 1 )0.99 ² + ( 5 - 1)1.15² / 5 + 5 + 5 - 3

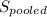`S_(pooled)`² = (4.9284 + 3.9204 + 5.29) / 12

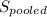`S_(pooled)`² = 14.1388 / 12

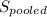`S_(pooled)`² = 1.1782

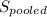`S_(pooled)` = √1.1782

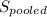`S_(pooled)` = 1.08544 ≈ 1.09

Therefore, The Pooled Standard Deviation for these data sets is 1.09