Answer:
Ri = 0.8 Ω
V= 2.55 V
Step-by-step explanation:
- Since the internal resistance of the battery is connected in series with the resistor connected across the battery's terminals, applying Ohm's Law, we can write the following equation, when R₁=4.30 Ω, and I₁=0.500A:

- We can apply exactly this same expression, when R₂ = 9.4Ω, and I₂ = 0.250A:
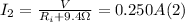
- Now, we can divide (1) by (2) as follows:
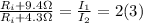
- Solving for Ri, we get Ri = 0.8 Ω
- Replacing this value in (1) we can solve for V, as follows:
- V = I₁ * (Ri + R₁) = 0.500 A * (0.8Ω + 4.3Ω) = 2.55 V
⇒ V = 2.55 V (emf of the battery)