Question

The cart travels the track again and now experiences a constant tangential acceleration from point A to point C. The speeds of the cart are 13.2 ft/s at point A and 17.4 ft/s at point C. The cart takes 3.00 s to go from point A to point C, and the cart takes 1.20 s to go from point B to point C. What is the cart's speed at point B

Answer 1

the cart's speed at point B is 15.72 ft/s

Step-by-step explanation:

Given the data in the question;

The car travels from point A to C in 3.00 s, its average acceleration
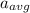`a_(avg)` will be;

`a_(avg)` = [
`V_(c)` -
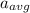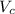`V_(A)`] / Δt

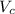`V_(c)` is 17.4 ft/s,
`V_(A)` is 13.2 ft/s and Δt is 3.00 s

so we substitute

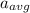`a_(avg)` = [17.4 - 13.2] / 3

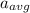`a_(avg)` = 4.2 / 3

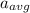`a_(avg)` = 1.4 ft/s²

so average acceleration of the cart between the points A and B is 1.4 ft/s²

The instantaneous value of the velocity of the cart at point B will be;

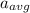`a_(avg)` = Δv / Δt

now substitute [
`V_(c)` -
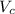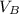`V_(B)`] for Δv and t' for Δt

`a_(avg)` = [
`V_(c)` -
`V_(B)`] / t'

`V_(B)` =
`V_(c)` -
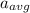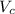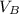`a_(avg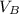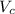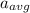)`( t' )

so we substitute 17.4 ft/s for
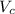`V_(c)`, 1.20 s for t' and
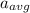`a_(avg)` = 1.4 ft/s²

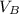`V_(B)` = 17.4 - (1.4 × 1.20)

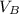`V_(B)` = 17.4 - 1.68

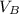`V_(B)` = 15.72 ft/s

Therefore, the cart's speed at point B is 15.72 ft/s