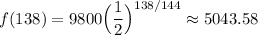Answer:
About 5043.58
Explanation:
The standard form for an exponential decay after t time is:
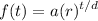
Where a is the initial value, r is the rate decay, t is the time that has passed, and d is the amount of time it takes for 1 cycle.
The initial value is 9800. So a = 9800.
The quantity cuts in half. So, r = 1/2.
And it cuts in half every 6 days. For this question, we will convert this to hours. 6 days = 144 hours. So, we can let d = 144, where t will be in hours.
Therefore, our function is:
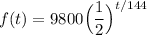
Where t is the amount of time that has passed, in hours.
Then the quantity left after 138 hours will be: