Final Answer:
The correct option is 1 A = 1.6 and C = 0.5.
Step-by-step explanation:
The general form of the equation for a vertical ellipse is given by
 . Using the provided points (3.75, 0), (0, 2.71), (1, -7), and (-1, -5.725), we can substitute these coordinates into the equation. Since the ellipse is vertical, the term with the coefficient of x^2 is retained.
. Using the provided points (3.75, 0), (0, 2.71), (1, -7), and (-1, -5.725), we can substitute these coordinates into the equation. Since the ellipse is vertical, the term with the coefficient of x^2 is retained.
Let's plug in the values:
1. For point (3.75, 0):
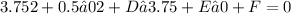
2. For point (0, 2.71):

3. For point (1, -7):
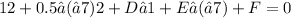
4. For point (-1, -5.725):
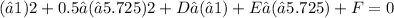
Solving this system of equations will yield the values for D, E, and F. Once these are obtained, the coefficients A and C can be identified. The correct solution is A = 1.6 and C = 0.5, matching option 1.