Question

A woman stands on a moving sidewalk (conveyor belt) that is moving to the right at a speed of 2 m/s relative to the ground. A dog runs on the belt toward the woman at a speed of 8 m/s relative to the belt. A conveyor belt is moving to the right at the speed v subscript belt, ground equals 2 meters per second. A girl stands stationary on the left end of the belt. A dog runs on the belt to the left toward the girl at the speed v subscript dog, belt equals 8 meters per second. What is the speed of the dog relative to the ground

Answer 1

The dog's velocity relative to the ground is 6 m/s to the left, obtained by subtracting the conveyor belt's velocity from the dog's velocity relative to the belt.

Step-by-step explanation:

The velocity of the dog relative to the ground is calculated by taking into account the velocity of the conveyor belt relative to the ground and the velocity of the dog relative to the conveyor belt. The conveyor belt moves to the right at 2 m/s, and the dog runs to the left (opposite to the belt's movement) at 8 m/s relative to the belt.

To find the dog's velocity relative to the ground, we subtract the belt's velocity from the dog's velocity, because they are moving in opposite directions:

Velocity of dog relative to the ground = Velocity of dog relative to belt - Velocity of belt relative to ground = 8 m/s - 2 m/s = 6 m/s to the left.

Answer 2

V_{2G} = - 10 m / s

Step-by-step explanation:

This is an exercise on relative velocities in one dimension, let's use the subscript 1 for the girl, the 2 for the but, the index 3 for the conveyor belt and the index G for the Earth

we will consider the velocity is positive to the right.

They indicate the speed of the belt with respect to the ground = 2 m/s

the dog speed with respect to the belt = - 8 m / s

Dog speed is requested with respect to the Earth

`V_(2G) = V_(23) - V_(3G)`

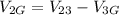
notice that the inner subscripts cancel out.

We calculate

= -8 - 2

V_{2G} = - 10 m / s

the negative sign indicates that the dog moves to the left