Question

PLEASE HELP !! 50 POINTS

Answer 1

`\textsf{ 2x + 4y = 6 $\longrightarrow $ Multiply by $\boxed{\; 3}$ on both sides}`

`\textsf{ 3x + 5y = 7 $\longrightarrow $ Multiply by $\boxed{\; 2}$ on both sides}`

Explanation:

To eliminate the x-terms in the system of equations
`\sf 2x + 4y = 6` and
`\sf 3x + 5y = 7`, we can manipulate the equations to make the coefficients of x in both equations the same or multiples of each other.

In this case, we want to eliminate the x-terms by making the coefficients the same.

First, let's find the least common multiple of 2 and 3, which is 6.

Multiply the first equation by 3 and the second equation by 2:

`\sf \begin{aligned} 3(2x + 4y) &= 3(6) \\ 2(3x + 5y) &= 2(7) \end{aligned}`

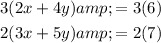
This results in the following system of equations:

`\sf \begin{aligned} 6x + 12y &= 18 \\ 6x + 10y &= 14 \end{aligned}`

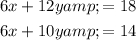
Now, the coefficients of x in both equations are the same (6).

We can subtract the second equation from the first to eliminate the x-terms:

`\sf \begin{aligned} (6x + 12y) - (6x + 10y) &= 18 - 14 \\ 2y &= 4 \end{aligned}`

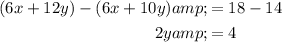
Now, we can solve for y by dividing both sides by 2:

`\sf y = 2`

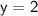
Now that we have the value for y, we can substitute it back into either of the original equations to solve for x. Let's use the first equation:

`\sf 2x + 4(2) = 6`

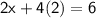
Solving for x:

`\sf 2x + 8 = 6`

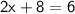
Subtracting 8 from both sides:

`\sf 2x = -2`

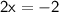
Dividing by 2:

`\sf x = -1`

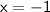
So, the solution to the system of equations is
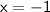`\sf x = -1` and
`\sf y = 2`.

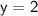Therefore, the answer is:

`\textsf{ 2x + 4y = 6 $\longrightarrow $ Multiply by $\boxed{\; 3}$ on both sides}`

`\textsf{ 3x + 5y = 7 $\longrightarrow $ Multiply by $\boxed{\; 2}$ on both sides}`